Class 12: Maths Chapter 9 solutions. Complete Class 12 Maths Chapter 9 Notes.
Contents
RD Sharma Solutions for Class 12 Maths Chapter 9–Continuity
RD Sharma 12th Maths Chapter 9, Class 12 Maths Chapter 9 solutions
Exercise 9.1 Page No: 9.16
1. Test the continuity of the following function at the origin:
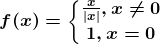
Solution:
Given
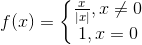
Consider LHL at x = 0
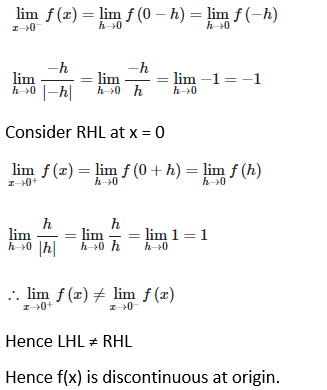
2. A function f(x) is defined as

Show that f(x) is continuous at x = 3.
Solution:
Given
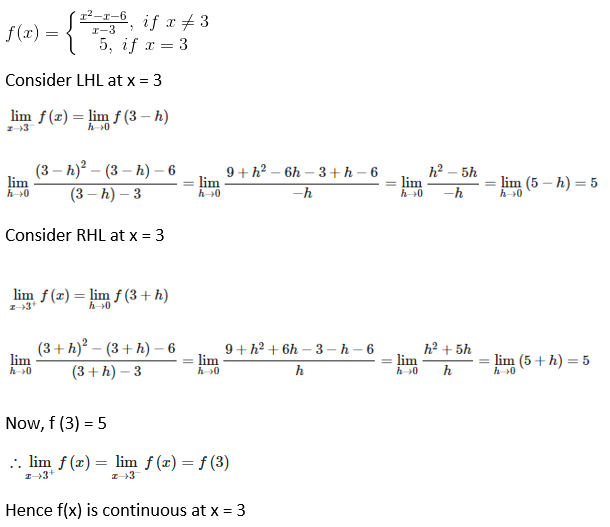
3. A function f(x) is defined as
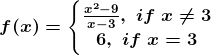
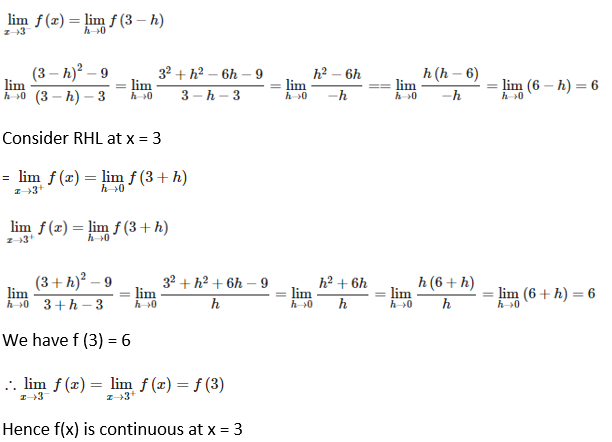
Show that f(x) is continuous at x = 3.
Solution:
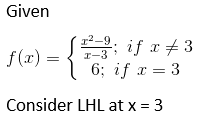
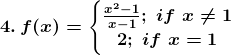
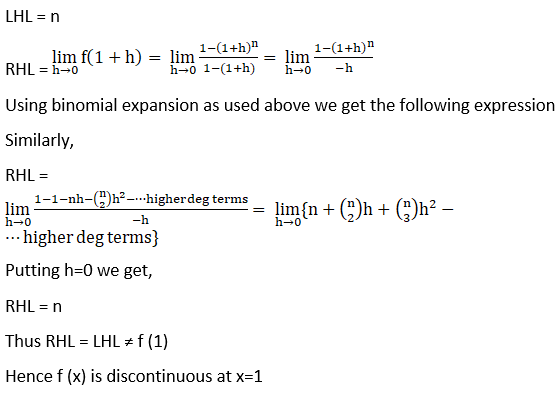
Find whether f(x) is continuous at x = 1.
Solution:
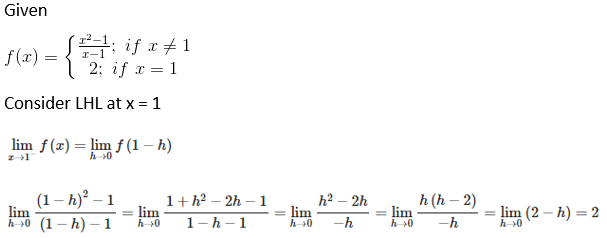
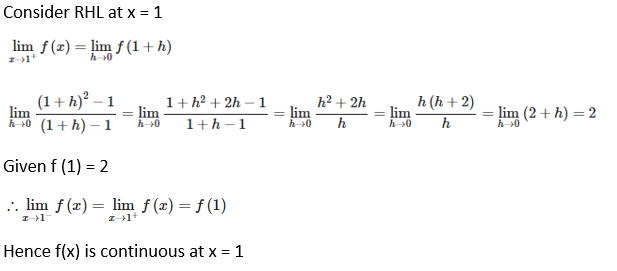
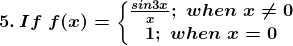
Find whether f(x) is continuous at x = 0.
Solution:


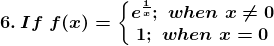
Find whether f(x) is continuous at x = 0.
Solution:
Given
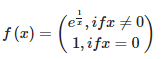
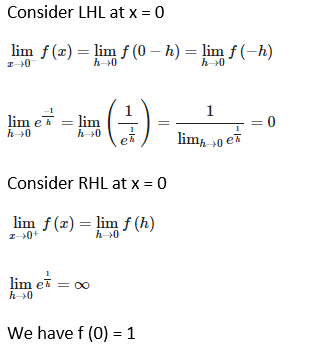

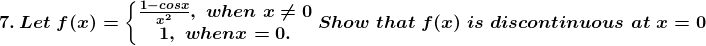
Solution:
Given
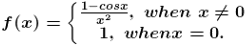
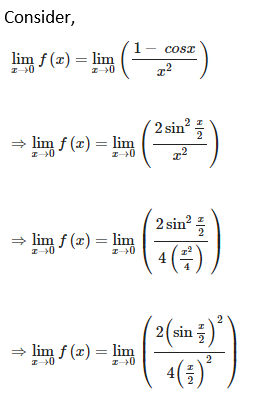
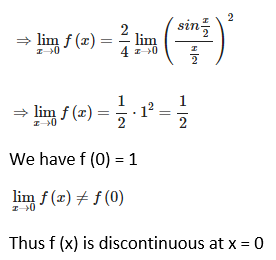
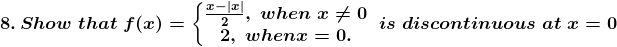
Solution:

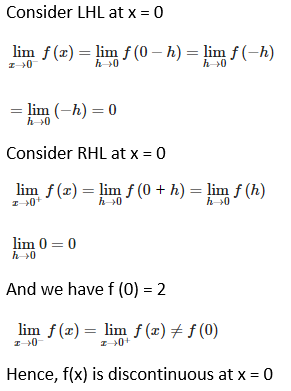

Solution:


10. Discuss the continuity of the following functions at the indicated point(s):
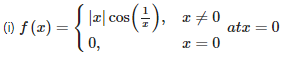
Solution:
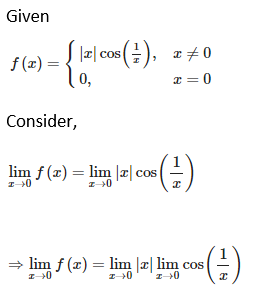

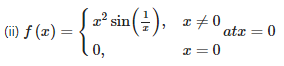
Solution:
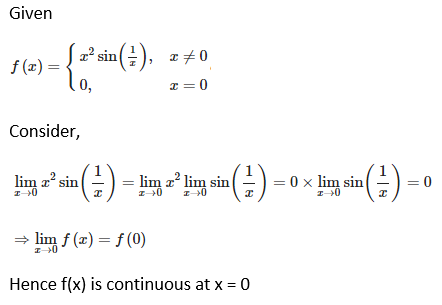
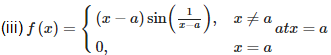
Solution:
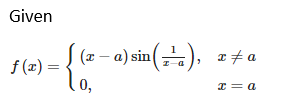
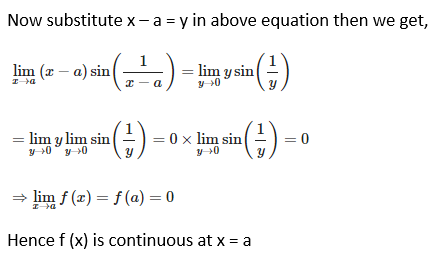
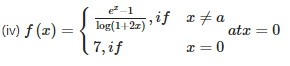
Solution:
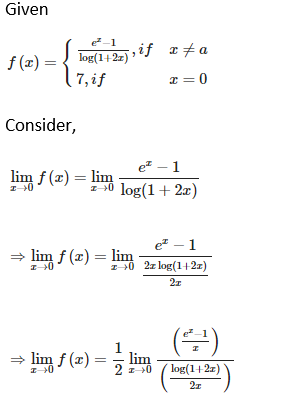
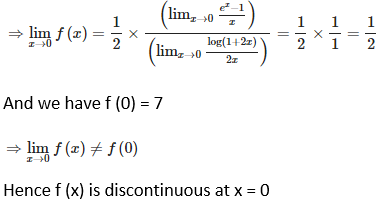
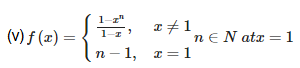
Solution:


Solution:
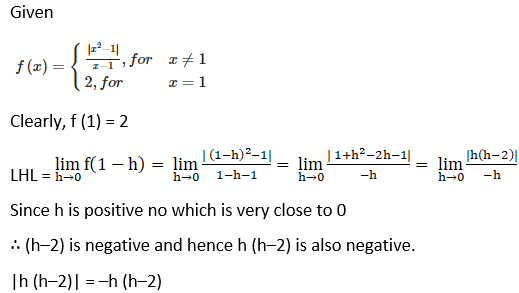

Solution:
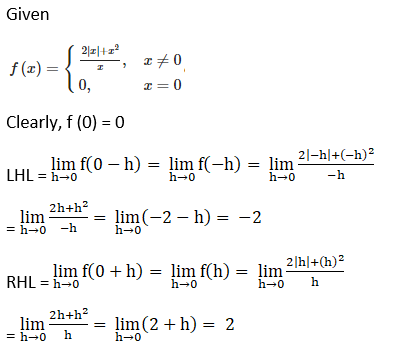

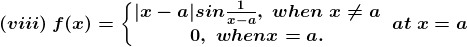
Solution:
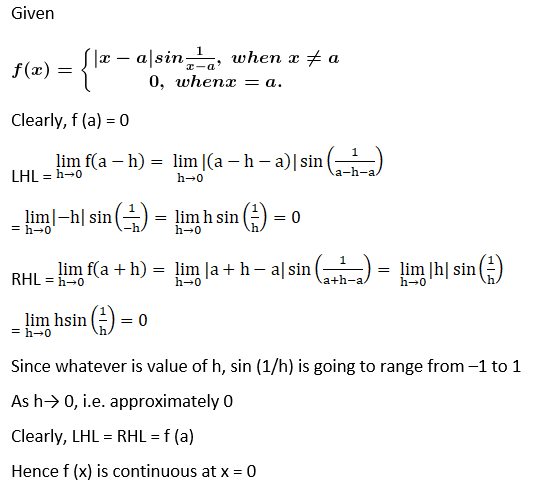
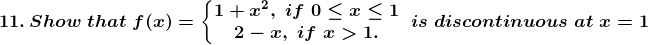
Solution:
Given

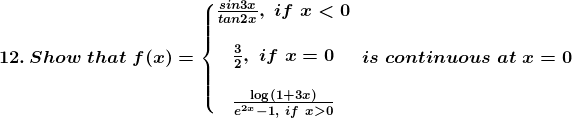
Solution:
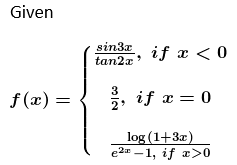
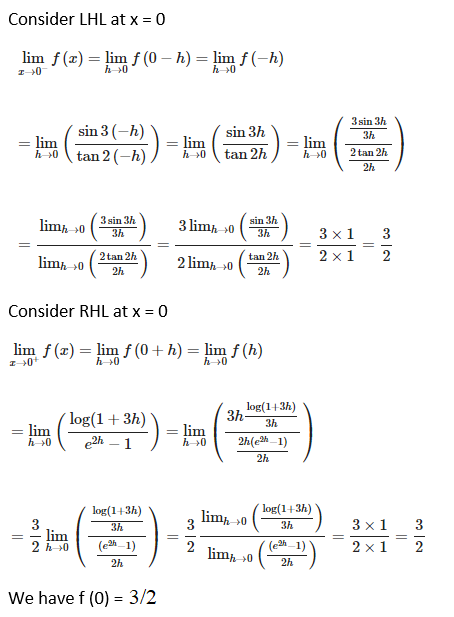
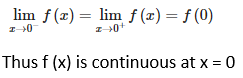
13. Find the value of a for which the function f defined by
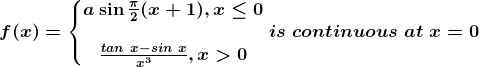
Solution:

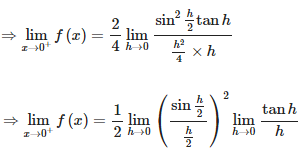
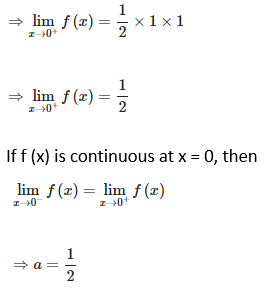
14. Examine the continuity of the function
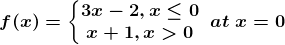
Also sketch the graph of this function.
Solution:
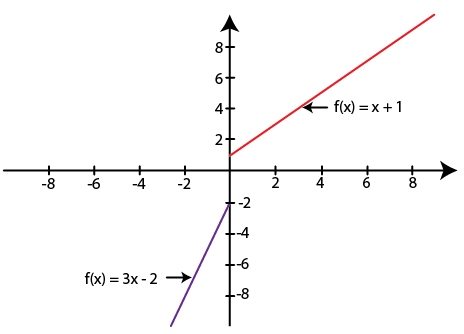

Hence f (x) is discontinuous at x = 0
15. Discuss the continuity of the function
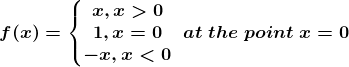
Solution:
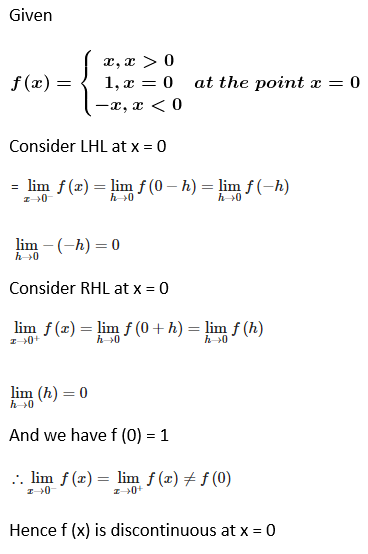
16. Discuss the continuity of the function
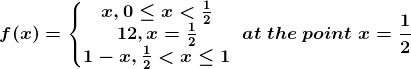
Solution:
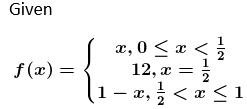

17. Discuss the continuity of
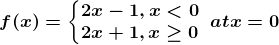
Solution:
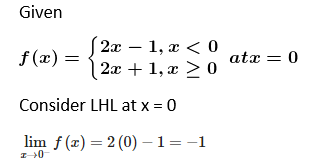
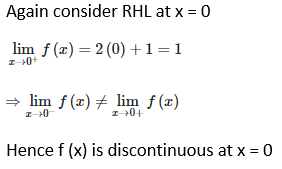
18. For what value of k is the function
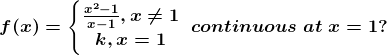
Solution:
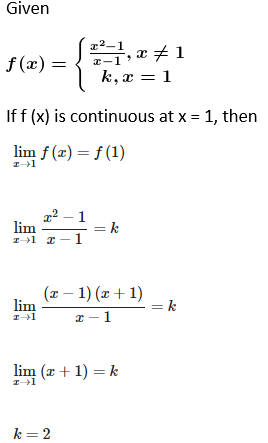
19. Determine the value of the constant k so that the function

Solution:
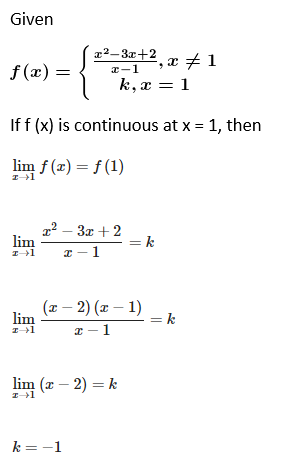
20. For what value of k is the function
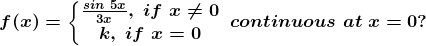
Solution:
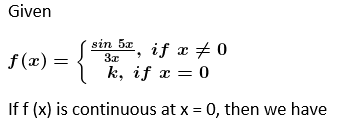
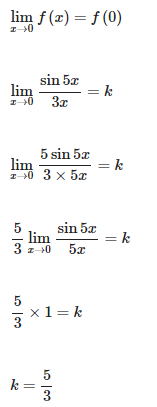
21. Determine the value of the constant k so that the function

Solution:
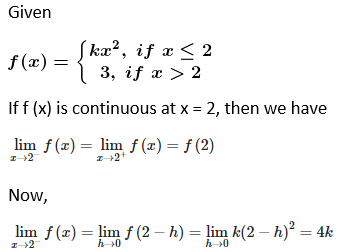
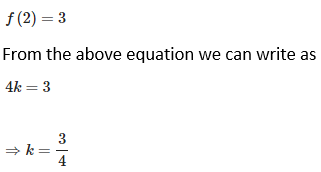
22. Determine the value of the constant k so that the function
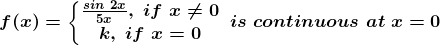
Solution:
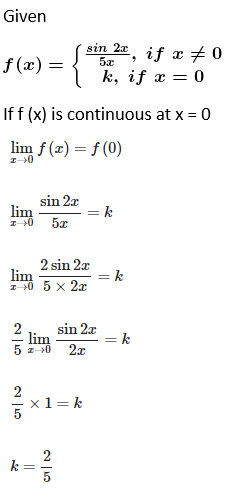
23. Find the values of a so that the function
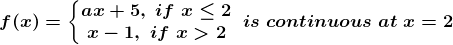
Solution:

Exercise 9.2 Page No: 9.34
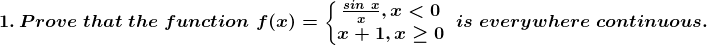
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
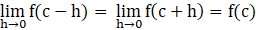
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
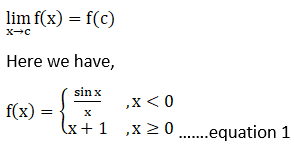
To prove it everywhere continuous we need to show that at every point in the domain of f(x) [domain is nothing but a set of real numbers for which function is defined]
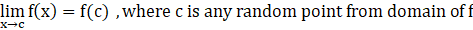
Clearly from definition of f(x), f(x) is defined for all real numbers.
Now we need to check continuity for all real numbers.
Let c is any random number such that c < 0 [thus c being a random number, it can include all negative numbers]
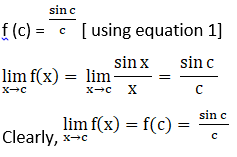
We can say that f(x) is continuous for all x < 0
Now, let m be any random number from the domain of f such that m > 0
Thus m being a random number, it can include all positive numbers]
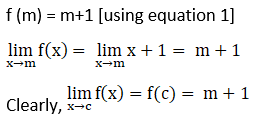
Therefore we can say that f(x) is continuous for all x > 0
As zero is a point at which function is changing its nature so we need to check LHL, RHL separately
f (0) = 0 + 1 = 1 [using equation 1]
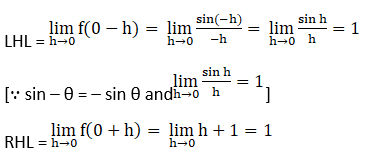
Thus LHL = RHL = f (0).
Therefore f (x) is continuous at x = 0
Hence, we proved that f is continuous for x < 0; x > 0 and x = 0
Thus f(x) is continuous everywhere.
Hence, proved.

Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
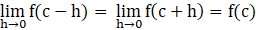
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
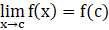
Here we have,
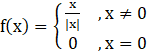
…….equation 1
The function is defined for all real numbers, so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)
Function is changing its nature (or expression) at x = 0, so we need to check its continuity at x = 0 first.
We know that from the definition of mod function we have
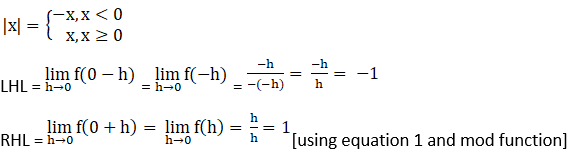
f (0) = 0 [using equation 1]
Clearly, LHL ≠ RHL ≠ f (0)
∴ Function is discontinuous at x = 0
Let c be any real number such that c > 0

Therefore f (x) is continuous everywhere for x > 0.
Let c be any real number such that c < 0
Therefore f (c) =
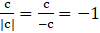
[Using equation 1 and idea of mod function]
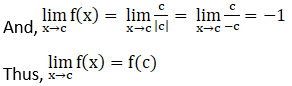
Therefore f (x) is continuous everywhere for x < 0.
Hence, we can conclude by stating that f (x) is continuous for all Real numbers except zero that is discontinuous at x = 0.
3. Find the points of discontinuity, if any, of the following functions:
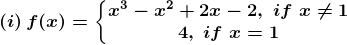
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
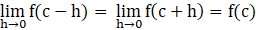
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
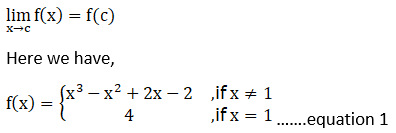
Function is defined for all real numbers so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)
Function is changing its nature (or expression) at x = 1, so we need to check its continuity at x = 1.
Clearly, f (1) = 4 [using equation 1]

∴ f (x) is discontinuous at x = 1.
Let c be any real number such that c ≠ 0
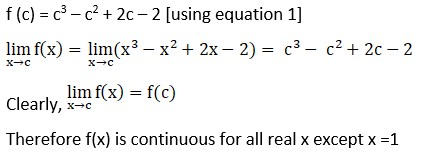
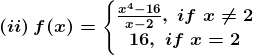
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
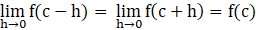
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
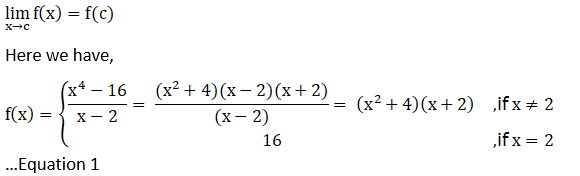
The function is defined for all real numbers, so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)
Function is changing its nature (or expression) at x = 2, so we need to check its continuity at x = 2 first.
Clearly, f (2) = 16 [from equation 1]
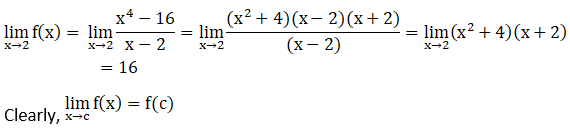
∴ f (x) is continuous at x = 2.
Let c be any real number such that c ≠ 0
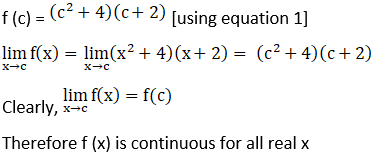
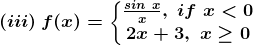
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
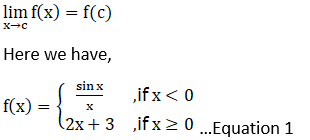
The function is defined for all real numbers, so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)
Let c is any random number such that c < 0 [thus c being a random number, it can include all negative numbers]
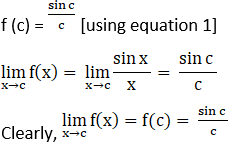
We can say that f(x) is continuous for all x < 0
Now, let m be any random number from the domain of f such that m > 0
Thus m being a random number, it can include all positive numbers]
f (m) = 2m + 3 [from equation 1]
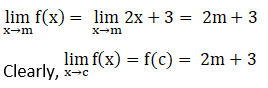
We can say that f(x) is continuous for all x > 0
As zero is a point at which function is changing its nature so we need to check LHL, RHL separately
f (0) = 2 × 0 + 3 = 3 [using equation 1]
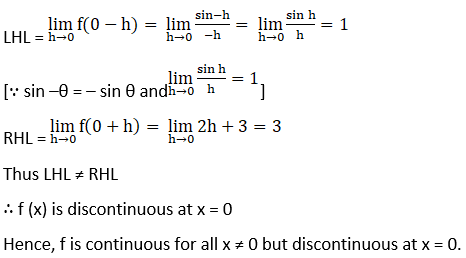
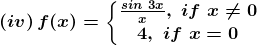
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
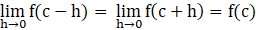
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if

The function is defined for all real numbers, so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)
Let c is any random number such that c ≠ 0 [thus c being a random number, it can include all numbers except 0]
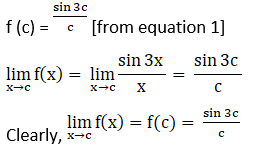
We can say that f(x) is continuous for all x ≠ 0
As zero is a point at which function is changing its nature, so we need to check the continuity here.
f (0) = 4 [using equation 1]
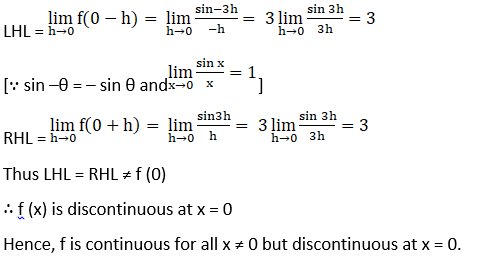
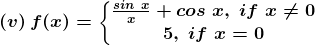
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
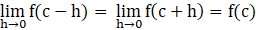
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
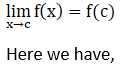
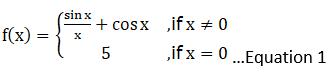
The function is defined for all real numbers, so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)
Let c is any random number such that c ≠ 0 [thus c being a random number, it can include all numbers except 0]
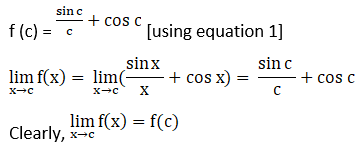
We can say that f(x) is continuous for all x ≠ 0
As zero is a point at which function is changing its nature, so we need to check the continuity here.
f (0) = 5 [using equation 1]


Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
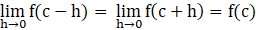
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
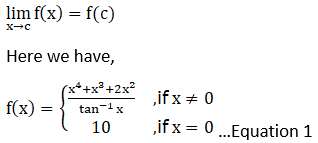
The function is defined for all real numbers, so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)
Let c is any random number such that c ≠ 0 [thus c being a random number, it can include all numbers except 0]
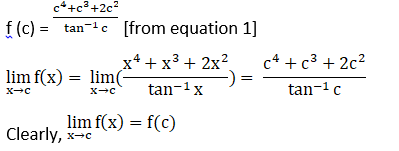
We can say that f(x) is continuous for all x ≠ 0
As zero is a point at which function is changing its nature so we need to check the continuity here.
f (0) = 10 [using equation 1]
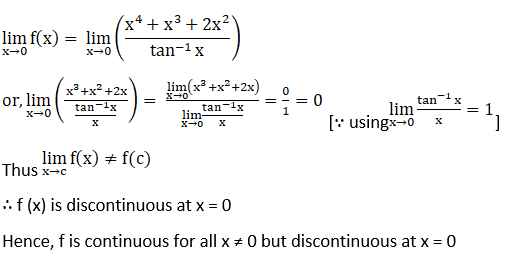
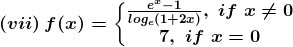
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
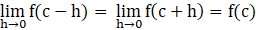
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
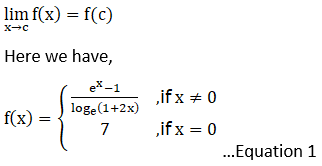
Function is defined for all real numbers so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)
Let c is any random number such that c ≠ 0 [thus c being random number, it is able to include all numbers except 0]
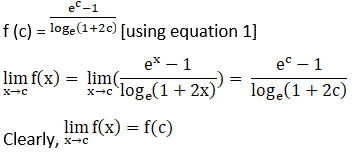
We can say that f(x) is continuous for all x ≠ 0
As x = 0 is a point at which function is changing its nature so we need to check the continuity here.
Since, f (0) = 7 [from equation 1]

Log (1+x) and ex in its Taylor form.
From sandwich theorem numerator and denominator conditions also hold for this limit

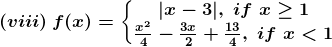
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
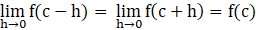
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if


Function is defined for all real numbers so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)
Let c is any random number such that c < 1 [thus c being a random number, it can include all numbers less than 1]
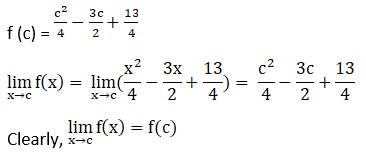
We can say that f(x) is continuous for all x < 1
As x = 1 is a point at which function is changing its nature, so we need to check the continuity here.
f (1) = | 1 – 3 | = 2 [from equation 1]
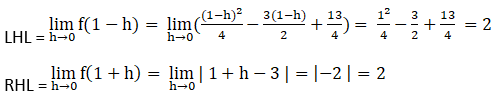
Thus LHL = RHL = f (1)
∴ f (x) is continuous at x = 1
Now, again f(x) is changing its nature at x = 3, so we need to check continuity at x = 3
f (3) = 3 – 3 = 0 [using equation 1]
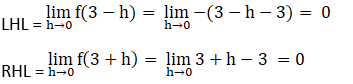
Thus LHL = RHL = f (3)
∴ f (x) is continuous at x = 3
For x > 3; f(x) = x–3 whose plot is linear, so it is continuous for all x > 3
Similarly, for 1 < x < 3, f(x) = 3 – x whose plot is again a straight line and thus continuous for all point in this range.
Hence, f(x) is continuous for all real x.
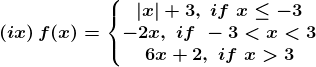
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if

Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
A function is continuous at x = c if
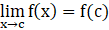
Similarly, we can define it for variable x, if x ≥ 0 |x| = x
If x < 0 |x| = (–x)
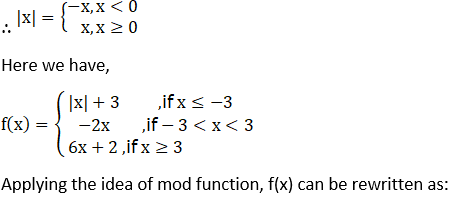
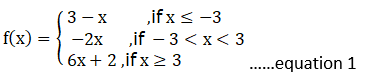
Function is defined for all real numbers so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)
Let c is any random number such that c < –3 [thus c being random number, it is able to include all numbers less than –3]
f (c) = 3 – c [from equation 1]

Thus LHL = RHL = f (–3)
∴ f (x) is continuous at x = –3
Let c is any random number such that –3 < m < 3 [thus c being random number, it is able to include all numbers between –3 and 3]
f (c) = -2m [ using equation 1]
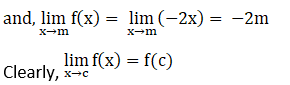
We can say that f(x) is continuous for all –3 < x < 3
Now, again f(x) is changing its nature at x = 3, so we need to check continuity at x = 3
f (3) = 6 × 3 + 2 = 20 [using equation 1]
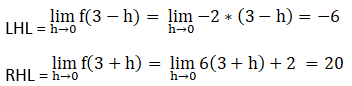
Thus LHL ≠ RHL
∴ f (x) is discontinuous at x = 3
For x > 3; f(x) = 6x + 2 whose plot is linear, so it is continuous for all x > 3
Hence, f(x) is continuous for all real x except x = 3
There is only one point of discontinuity at x = 3
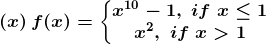
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
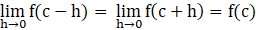
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
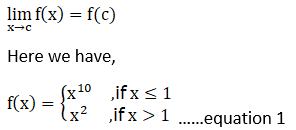
Function is defined for all real numbers so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)
Let c is any random number such that c < 1 [thus c being random number, it is able to include all numbers less than 1]
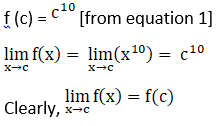
We can say that f(x) is continuous for all x < 1
As x = 1 is a point at which function is changing its nature so we need to check the continuity here.
f (1) = 110 = 1 [using equation 1]

Hence, f(x) is continuous for all real x
There no point of discontinuity. It is everywhere continuous

Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
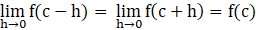
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
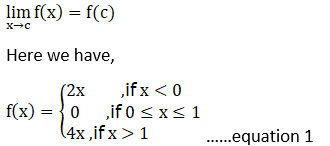
The function is defined for all real numbers, so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)
Let c is any random number such that c < 0 [thus c being a random number, it can include all numbers less than 0]
f (c) = 2c
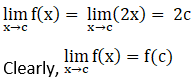
We can say that f(x) is continuous for all x < 0
As x = 0 is a point at which function is changing its nature, so we need to check the continuity here.
f (0) = 0 [using equation 1]
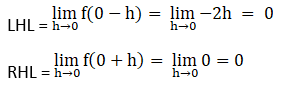
Thus LHL = RHL = f (0)
∴ f (x) is continuous at x = 0
Let m is any random number such that 0 < m < 1 [thus m being a random number, it can include all numbers greater than 0 and less than 1]
f (m) = 0 [using equation 1]
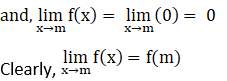
We can say that f(x) is continuous for all 0 < x < 1
As x = 1 is again a point at which function is changing its nature, so we need to check the continuity here.
f (1) = 0
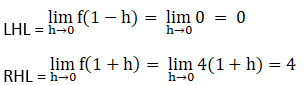
∴ f (x) is discontinuous at x = 1
Let k is any random number such that k > 1 [thus k being a random number, it can include all numbers greater than 1]
f (k) = 4k [using equation 1]

We can say that f(x) is continuous for all x > 1
Hence, f(x) is continuous for all real value of x, except x =1
There is a single point of discontinuity at x = 1

Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
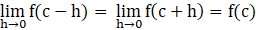
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
This is very precise, using our fundamental idea of limit from class 11 we can summarise it as, A function is continuous at x = c if
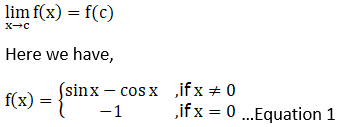
Function is defined for all real numbers so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)
Let c is any random number such that c ≠ 0 [thus c being a random number, it can include all numbers except 0]
f (c) = sin c – cos c [using equation 1]
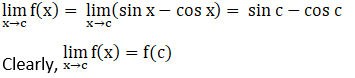
We can say that f(x) is continuous for all x ≠ 0
As zero is a point at which function is changing its nature, so we need to check the continuity here.
f (0) = –1 [using equation 1]

∴ f (x) is continuous at x = 0
Hence, f is continuous for all x.
f (x) is continuous everywhere.
No point of discontinuity.
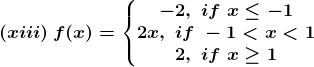
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
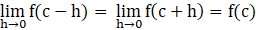
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
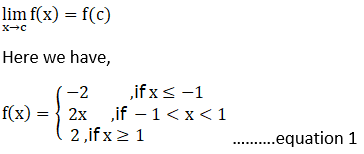
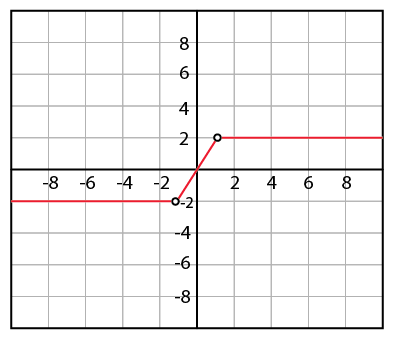
Function is defined for all real numbers so we need to comment about its continuity for all numbers in its domain (domain = set of numbers for which f is defined)
For x < –1, f(x) is having a constant value, so the curve is going to be straight line parallel to x–axis.
So, it is everywhere continuous for x < –1.
Similarly for –1 < x < 1, plot on X–Y plane is a straight line passing through origin.
So, it is everywhere continuous for –1 < x < 1.
And similarly for x > 1, plot is going to be again a straight line parallel to x–axis
∴ it is also everywhere continuous for x > 1
As x = –1 is a point at which function is changing its nature so we need to check the continuity here.
f (–1) = –2
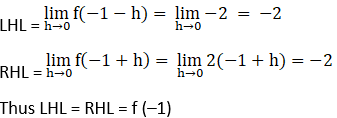
∴ f (x) is continuous at x = –1
Also at x = 1 function is changing its nature so we need to check the continuity here too.
f (1) = 2 [using equation 1]
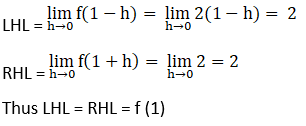
∴ f (x) is continuous at x = 1
Thus, f(x) is continuous everywhere and there is no point of discontinuity.
4. In the following, determine the value(s) of constant(s) involved in the definition so that the given function is continuous:
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
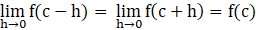
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
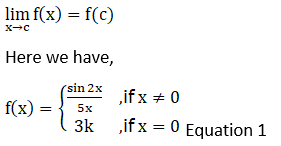
Function is defined for all real numbers and we need to find the value of k so that it is continuous everywhere in its domain (domain = set of numbers for which f is defined)
As, for x ≠ 0 it is just a combination of trigonometric and linear polynomial both of which are continuous everywhere.
As x = 0 is only point at which function is changing its nature so it needs to be continuous here.
f (0) = 3k [using equation 1]
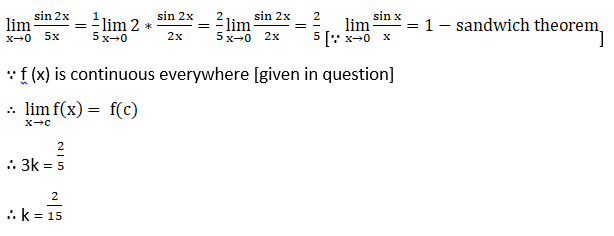

Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
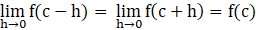
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
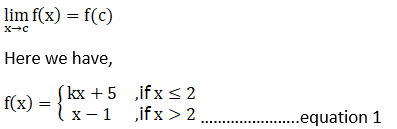
To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.
As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere.
From equation 1, it is clear that f(x) is changing its expression at x = 2
Given, f (x) is continuous everywhere

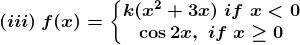
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
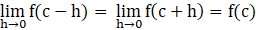
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
This is very precise, using our fundamental idea of limit from class 11 we can summarise it as, A function is continuous at x = c if
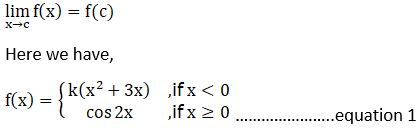
To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.
As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere
From equation 1, it is clear that f(x) is changing its expression at x = 0
Given, f (x) is continuous everywhere
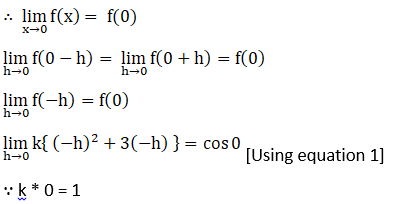
As above equality never holds true for any value of k
k = not defined
No such value of k is possible for which f(x) is continuous everywhere.
f (x) will always have a discontinuity at x = 0

Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
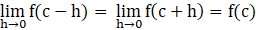
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
This is very precise, using our fundamental idea of limit from class 11 we can summarise it as, A function is continuous at x = c if
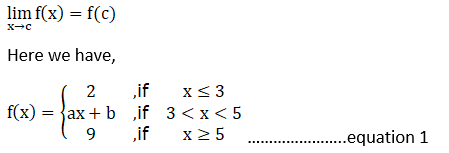
To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.
As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere
From equation 1, it is clear that f(x) is changing its expression at x = 3
Given, f(x) is continuous everywhere
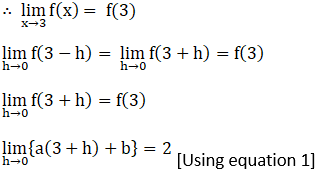
∴ 3a + b = 2 ……………….Equation 2
Also from equation 1, it is clear that f(x) is also changing its expression at x = 5
Given, f(x) is continuous everywhere

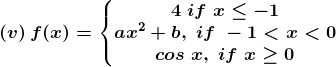
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
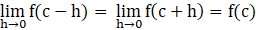
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if

To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.
As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere
From equation 1, it is clear that f(x) is changing its expression at x = –1
Given, f(x) is continuous everywhere
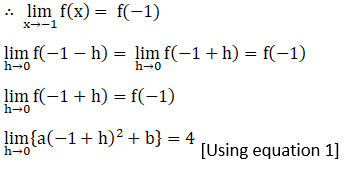
∴ a + b = 4 ……………….Equation 2
Also from equation 1, it is clear that f(x) is also changing its expression at x = 0
Given, f (x) is continuous everywhere
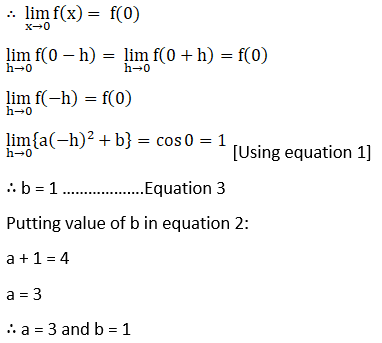
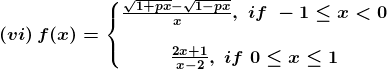
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
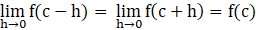
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.
As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere
From equation 1, it is clear that f(x) is changing its expression at x = 0
Given, f(x) is continuous everywhere
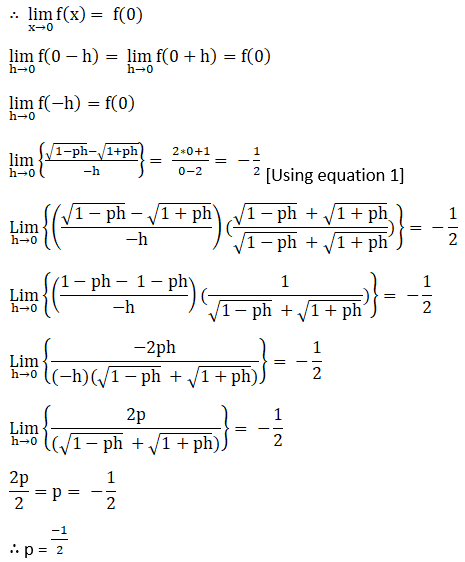
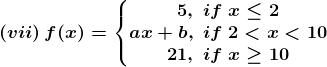
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
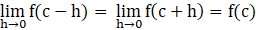
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
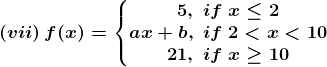
To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.
As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere
From equation 1, it is clear that f(x) is changing its expression at x = 2
Given, f(x) is continuous everywhere
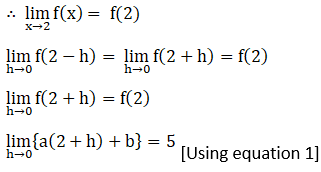
∴ 2a + b = 5 ……………….Equation 2
Also from equation 1, it is clear that f(x) is also changing its expression at x = 10
Given, f(x) is continuous everywhere
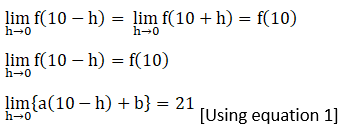
∴ 10a + b = 21 ……………….Equation 3
As, b = 21 – 10a
Putting value of b in equation 2, we get
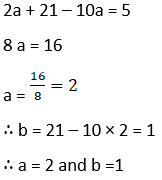
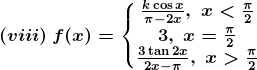
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
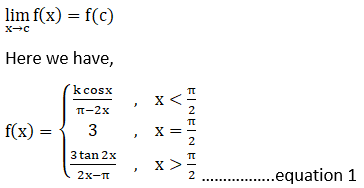
To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.
As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere
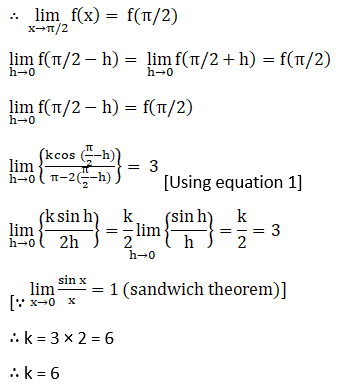
From equation 1, it is clear that f(x) is changing its expression at x = π/2
Given, f(x) is continuous everywhere

Is continuous on [0, ∞). Find the most suitable values of a and b.
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
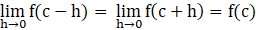
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if

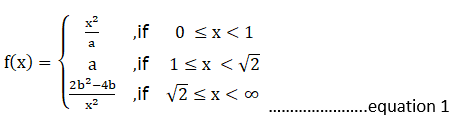
The function is defined for [0, ∞) and we need to find the value of a and b so that it is continuous everywhere in its domain (domain = set of numbers for which f is defined)
To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.
As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere
From equation 1, it is clear that f(x) is changing its expression at x = 1
Given, f (x) is continuous everywhere
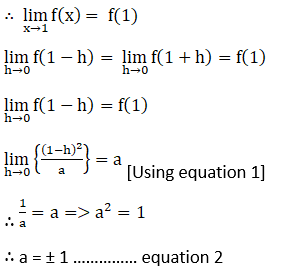
Also from equation 1, it is clear that f(x) is also changing its expression at x = √2
Given, f (x) is continuous everywhere
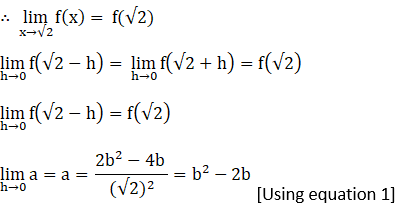
∴ b2 – 2b = a ……………….Equation 3
From equation 2, a = –1
b2 – 2b = –1
⇒ b2 – 2b + 1 = 0
⇒ (b – 1)2 = 0
∴ b = 1 when a = –1
Putting a = 1 in equation 3:
b2 – 2b = 1
⇒ b2 – 2b – 1 = 0
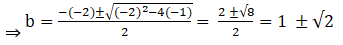
Thus,
For a = –1; b = 1
For a = 1; b = 1 ± √2
6. Find the values of a and b so that the function f (x) defined by
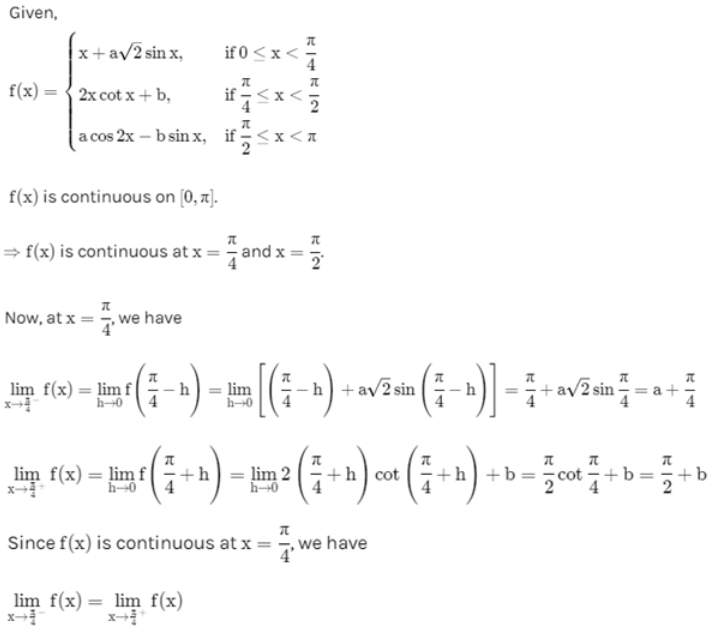
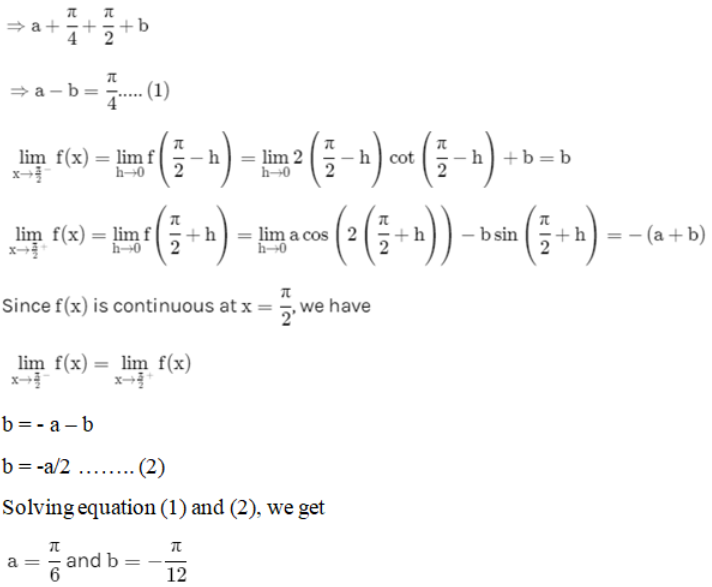
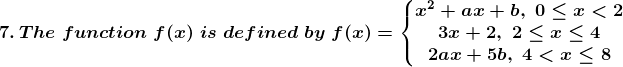
If f is continuous on [0, 8], find the values of a and b.
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
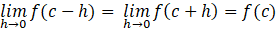
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
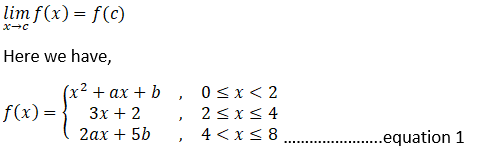
Function is defined for [0, 8] and we need to find the value of a and b so that it is continuous everywhere in its domain (domain = set of numbers for which f is defined)
To find the value of constants always try to check continuity at the values of x for which f(x) is changing its expression.
As most of the time discontinuities are here only, if we make the function continuous here, it will automatically become continuous everywhere
From equation 1, it is clear that f(x) is changing its expression at x = 2
Given, f (x) is continuous everywhere
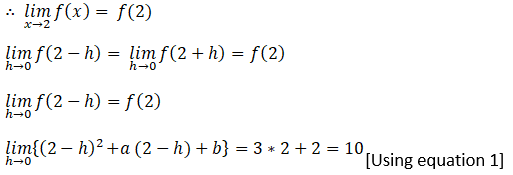
4+2a + b = 8
∴ 2a + b = 4
∴ b = 4 – 2a …………… equation 2
Also from equation 1, it is clear that f(x) is also changing its expression at x = 4
Given, f (x) is continuous everywhere
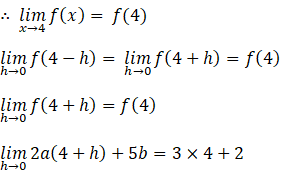
∴ 8a + 5b = 14 ……………….Equation 3
Putting value of a from equation 2 to equation 3
∴ 8a + 5(4–2a) = 14
⇒ 2a = 6
∴ a = 6/2
= 3
∴ b = 4 – 2×3 = –2
Thus, a = 3 and b = –2
8. If for x ≠ π/4, find the value which can be assigned to f (x) at x = π/4 so that the function f (x) becomes continuous everywhere in [0, π/2].
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
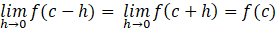
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if
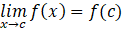
Function is defined for [0, π] and we need to find the value of f(x) so that it is continuous everywhere in its domain (domain = set of numbers for which f is defined)
As we have expression for x ≠ π/4, which is continuous everywhere in [0, π], so
If we make it continuous at x = π/4 it is continuous everywhere in its domain.


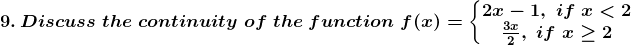
Solution:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if
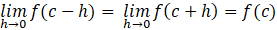
Where h is a very small positive number. i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
A function is continuous at x = c if

Function is changing its nature (or expression) at x = 2, so we need to check its continuity at x = 2 first.
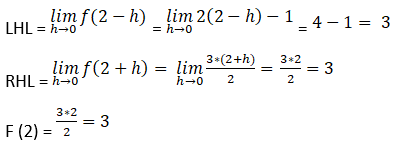
Clearly, LHL = RHL = f (2)
∴ Function is continuous at x = 2
Let c be any real number such that c > 2
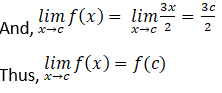
∴ f (x) is continuous everywhere for x > 2.
Let m be any real number such that m < 2
∴ f (m) = 2m – 1 [using equation 1]
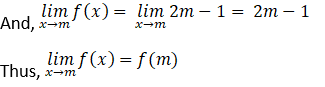
∴ f (x) is continuous everywhere for x < 2.
Hence, we can conclude by stating that f(x) is continuous for all Real numbers
RD Sharma Solutions for Class 12 Maths Chapter 9: Download PDF
RD Sharma Solutions for Class 12 Maths Chapter 9–Continuity
Download PDF: RD Sharma Solutions for Class 12 Maths Chapter 9–Continuity PDF
Chapterwise RD Sharma Solutions for Class 12 Maths :
- Chapter 1–Relation
- Chapter 2–Functions
- Chapter 3–Binary Operations
- Chapter 4–Inverse Trigonometric Functions
- Chapter 5–Algebra of Matrices
- Chapter 6–Determinants
- Chapter 7–Adjoint and Inverse of a Matrix
- Chapter 8–Solution of Simultaneous Linear Equations
- Chapter 9–Continuity
- Chapter 10–Differentiability
- Chapter 11–Differentiation
- Chapter 12–Higher Order Derivatives
- Chapter 13–Derivatives as a Rate Measurer
- Chapter 14–Differentials, Errors and Approximations
- Chapter 15–Mean Value Theorems
- Chapter 16–Tangents and Normals
- Chapter 17–Increasing and Decreasing Functions
- Chapter 18–Maxima and Minima
- Chapter 19–Indefinite Integrals
About RD Sharma
RD Sharma isn’t the kind of author you’d bump into at lit fests. But his bestselling books have helped many CBSE students lose their dread of maths. Sunday Times profiles the tutor turned internet star
He dreams of algorithms that would give most people nightmares. And, spends every waking hour thinking of ways to explain concepts like ‘series solution of linear differential equations’. Meet Dr Ravi Dutt Sharma — mathematics teacher and author of 25 reference books — whose name evokes as much awe as the subject he teaches. And though students have used his thick tomes for the last 31 years to ace the dreaded maths exam, it’s only recently that a spoof video turned the tutor into a YouTube star.
R D Sharma had a good laugh but said he shared little with his on-screen persona except for the love for maths. “I like to spend all my time thinking and writing about maths problems. I find it relaxing,” he says. When he is not writing books explaining mathematical concepts for classes 6 to 12 and engineering students, Sharma is busy dispensing his duty as vice-principal and head of department of science and humanities at Delhi government’s Guru Nanak Dev Institute of Technology.
